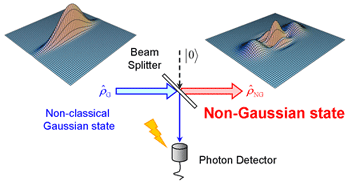
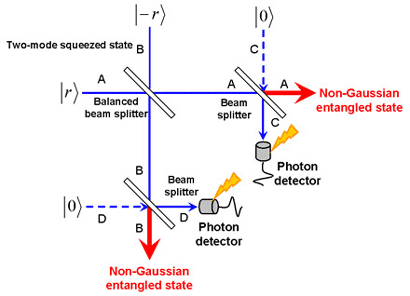
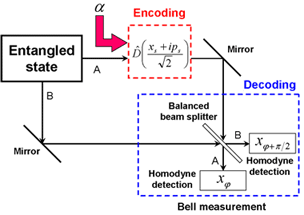
One of the fundamental topics of quantum information theory is how to transmit classical alphabets through a quantum limited channel. This subject includes the optimization problem of quantum signal detection, the investigations of the physical models realizing optical detection strategies, and the quantum coding theory which is nothing but quantum information processing with quantum information processing with quantum signal detection.
(a) Quantum signal detection
Quantum siglan detection is mathematically described by a positive-operator valued measure (POVM). The problem here is to find POVMs that optimize the figure of merits, such as minimizing the average error probability of discrimination, maximizing the mutual information for an ensemble of quantum states, and minimizing the Bayes cost of the identification of codes and more complex pattern structures. Physical realization by quantum circuit synthesis of optimal POVMs is also our important concern.
< Selected publications >
"Quantum channels showing superadditivity in capacity," M. Sasaki et al., Phys. Rev. A 58, 159 (1998); quant-ph/9801012.
"Accessible information for real symmetrical quantum sources," M. Sasaki et al., Phys. Rev. A 59, 3325 (1999); quant-ph/9812062.
"Quantum learning and universal quantum matching machine," M. Sasaki and A. Carlini, Phys. Rev. A 66, 022303 (2002);quant-ph/0202173.
"Optimal phase estimation and square root measurement," M. Sasaki, A. Carlini, and A. Chefles, J. Phys. A: Math. Gen. 34, 7017 (2001); quant-ph/0011057.
"Squeezing Quantum Information through a Classical Channel: Measuring the Quantumness" of a Set of Quantum States ," C. A. Fuchs and M. Sasaki, Quant. Inf. Comput. 3(5), 377 (2003); quant-ph/0107024.
(b) Implementation of quantum measurement via linear optics and photon counting
Linear optics and photon counters are powerful and currently (or near future) available devices to realize sophisticated quantum measurement for optical quantum signals. Our goal is to clarify, in principle, which class of quantum measurements can or can not be achieved by using only these devices.
< Selected publication >
"Implementation of projective measurements with linear optics andcontinuous photon counting," M. Takeoka, M. Sasaki, P. van Loock, and N. Luetkenhaus, Phys. Rev. A. 71, 022318 (2005); quant-ph/0410133.
(c) Quantum source coding
This topic aims at the development of efficient methods for expressing information using quantum states. In practice, this topic is related to the compression of quantum data (see Quantum Communication).
(d) Quantum channel coding
This topic consists of the development of methods for correctly transmitting information using quantum states. In practice, this topic is related to error correction (see Quantum Communication).